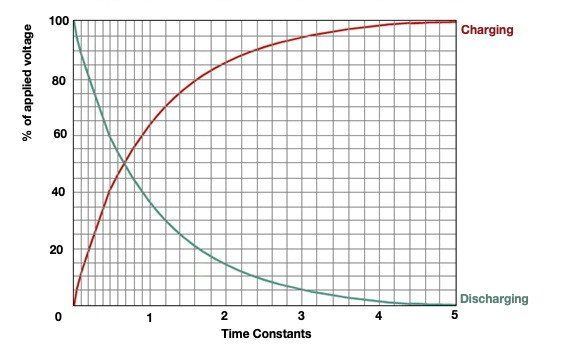
When you put a voltage across a capacitor, current will flow into the capacitor and the voltage across the capacitor will increase until the voltage across it reaches the value of the supply voltage. This is not a linear function. By that I mean that the voltage will increase quite rapidly at first, but the rate of increase will slow as time goes on.
Consider the simple circuit shown below. The “time constant” for this circuit is equal to the resistance in the circuit times the capacitance, or simply R × C. A capacitor charges capacitor charged to 63.2% of the applied voltage or discharges to 36.8% of the starting voltage after one time constant. After two time constants, a capacitor charges to 86.5% of the applied voltage, or discharges to 13.5% of the starting voltage. After three time constants, a capacitor is charged up to 95% of the applied voltage or discharged to 5% of the starting voltage.
QUESTION: What is the term for the time required for the capacitor in an RC circuit to be charged to 63.2% of the applied voltage or to discharge to 36.8% of its initial voltage? (E5B01)
ANSWER: One time constant
ANSWER: One time constant
QUESTION: What is the time constant of a circuit having two 220 microfarad capacitors and two 1 megohm resistors, all in parallel? (E5B04)
ANSWER: 220 seconds
ANSWER: 220 seconds
The equivalent resistance of two 1 MΩ resistors in parallel is 500 kΩ. The equivalent capacitance of two 220 μF capacitors in parallel is 440 μF. The time constant is R × C = 440×10-6 × 500×103 = 220 s.
Phase relationships
In an AC circuit, with only resistors, the voltage and current are in phase. What that means is that the voltage and current change in lock step. When the voltage increases, the current increases. When the voltage decreases, the current decreases.
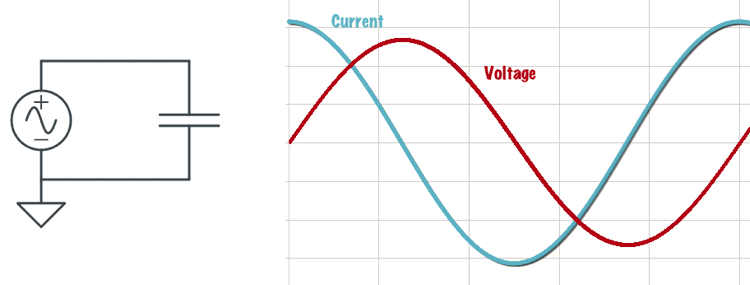
When there are capacitors and inductors in an AC circuit, however, the phase relationship between the voltage and current changes. Specifically, when an AC voltage is applied to a capacitor, the current begins to change, that is to increase and decrease, a quarter cycle, or 90 degrees, before the voltage changes. We say, therefore, that the current “leads” the voltage by 90 degrees. Conversely, we could also say that the voltage lags the current by 90 degrees. See figure below.
The current leads the current in an AC circuit with a capacitive reactance.
QUESTION: What is the relationship between the AC current through a capacitor and the voltage across a capacitor? (E5B09)
ANSWER: Current leads voltage by 90 degrees
ANSWER: Current leads voltage by 90 degrees
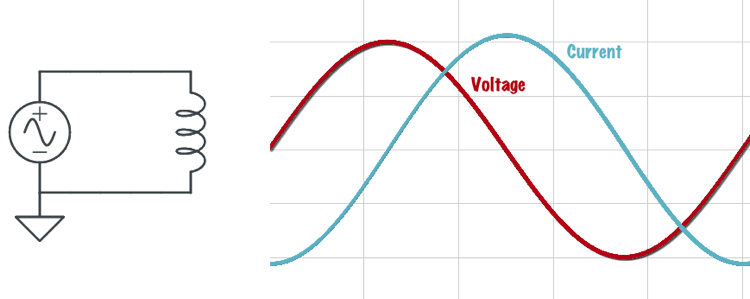
The relationship between the current through an inductor and the voltage across an inductor is exactly the opposite. That is to say that the voltage across an inductor increases and decreases before the current through the inductor increases and decreases. In an inductor, the voltage leads the current by a quarter cycle, meaning that the voltage leads current by 90 degrees. We could also say that the current lags the voltage. See figure below.
The voltage leads the current in a circuit with inductive reactance.
QUESTION: What is the relationship between the AC current through an inductor and the voltage across an inductor? (E5B10)
ANSWER: Voltage leads current by 90 degrees
ANSWER: Voltage leads current by 90 degrees
When there are resistors as well as a capacitor or inductor or both in a circuit, the relationship is a little more complicated. Let’s look at what happens in the series RLC circuit shown below.
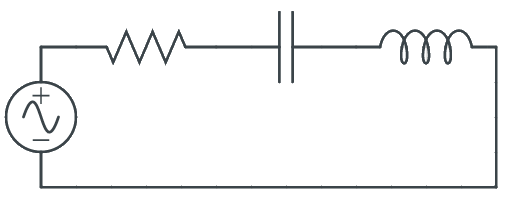
A series RLC circuit contains an inductive reactance, a capacitive reactance, and a resistance all in series.
In this circuit, there is resistance, capacitive reactance, and inductive reactance. The reactances subtract from one another. If the capacitive reactance is greater than the inductive reactance, the net reactance will be capacitive. If the inductive reactance is greater than the capacitive reactance, the net reactance will be inductive.
The resistance and the reactance add to one another, but they add vectorially. The reason for this is that the reactance will be 90 degrees out of phase with the resistance. This is shown in the figure below.
The magnitude of the impedance, Z, will be equal to √(R2 + X2) and the tangent of the phase angle will be equal to X/R. Let’s see how this works in several examples.
QUESTION: What is the phase angle between the voltage across and the current through a series RLC circuit if XC is 500 ohms, R is 1 kilohm, and XL is 250 ohms? (E5B07)
ANSWER: 14.0 degrees with the voltage lagging the current
Here’s how to calculate that. First, the total reactance, X = XC – XL = 250 Ω (capacitive). The phase angle = tan-1 (250/1000) = 14 degrees, and because the reactance is capacitive, the voltage will lag the current.
QUESTION: What is the phase angle between the voltage across and the current through a series RLC circuit if XC is 100 ohms, R is 100 ohms, and XL is 75 ohms? (E5B08)
ANSWER: 14 degrees with the voltage lagging the current
X = XC – XL = 25 Ω (capacitive). The phase angle = tan-1 (25/100) = 14 degrees, and because the reactance is capacitive, the voltage lags the current.
QUESTION: What is the phase angle between the voltage across and the current through a series RLC circuit if XC is 25 ohms, R is 100 ohms, and XL is 50 ohms? (E5B11)
ANSWER: 14 degrees with the voltage leading the current
X = XL – XC = 25 Ω (inductive). The phase angle = tan-1 (25/100) = 14 degrees, and because the reactance is inductive, the voltage leads the current.
Susceptance and admittance
While we most often work with reactances and impedances in amateur radio, in some cases, it’s more advantageous to work with susceptance and admittance. Admittance is the inverse of impedance and is a complex quantity. That is to say it has both real and imaginary components. The unit of admittance is the siemens (S).
QUESTION: What is admittance? (E5B12)
ANSWER: The inverse of impedance
QUESTION: How is impedance in polar form converted to an equivalent admittance? (E5B03)
ANSWER: Take the reciprocal of the magnitude and change the sign of the angle
Susceptance is the imaginary part of admittance, and the unit of susceptance is the siemens. B is the letter is commonly used to represent susceptance.
QUESTION: What is susceptance? (E5B06)
ANSWER: The imaginary part of admittance
QUESTION: What letter is commonly used to represent susceptance? (E5B02)
ANSWER: B
QUESTION: What happens to the magnitude of a pure reactance when it is converted to a susceptance? (E5B05)
ANSWER: It becomes the reciprocal
No comments:
Post a Comment